Texas Hold Em Ranking
- Sep 08, 2020 There is no “3 pair” hand rank in poker. When playing Texas Hold’em it’s technically possible to have three pairs, but since a poker hand only consists of 5 cards only the 2 highest pairs are in play. For example, if you hold Q-J and the board reads Q-J-6-A-A you only have two pair: Aces and Queens. Does Royal Flush have to be spades?
- Texas Hold'em Hand Rank. Hand Rank FAQ: Quiz. Learning the rank of hands in Texas Hold'em is just as important as learning the rules themselves. Be sure to know what beats what, so you won't be surprised the next time that flush beats your straight.
Poker is an exciting game of luck and sheer skills. In Poker, each player creates a set of five playing’s, i.e., Poker hands. Each Poker hand in the game has a Poker hand ranking that is compared against the competitor’s rank in order to decide who is the winner. In high games like Texas Holdem and Seven-card stud the Texas Holdem winning hand emerges as the champion. According to the Poker hand rules, there are ten hand rankings that determine the ultimate winner against the others.
Poker Hand Rankings In Texas Hold’em, you’ll receive two hole cards, which are the hidden cards only used by you. Throughout the course of the game, five more cards will be dealt into the middle of the table, and they’re called community cards. These community cards can be used by everyone at the table.

Learning about Poker hands and Texas Holdem winning hands will make you efficient enough to play your best hand and emerge as a champion. Make the most of your skills and opportunities and create a winning Poker hand on your next game at BLITZPOKER.
These are standard hand rankings for most poker games and apply to all high-hand poker variations including Texas holdem
You’ll find a printable poker hand rankings chart below the hand rankings as well as answers to some of the most frequently asked poker hand ranking
Do you think you have got what it win at Texas Holdem? You can always test your skills online. All good poker sites also has play without having to risk your own money.
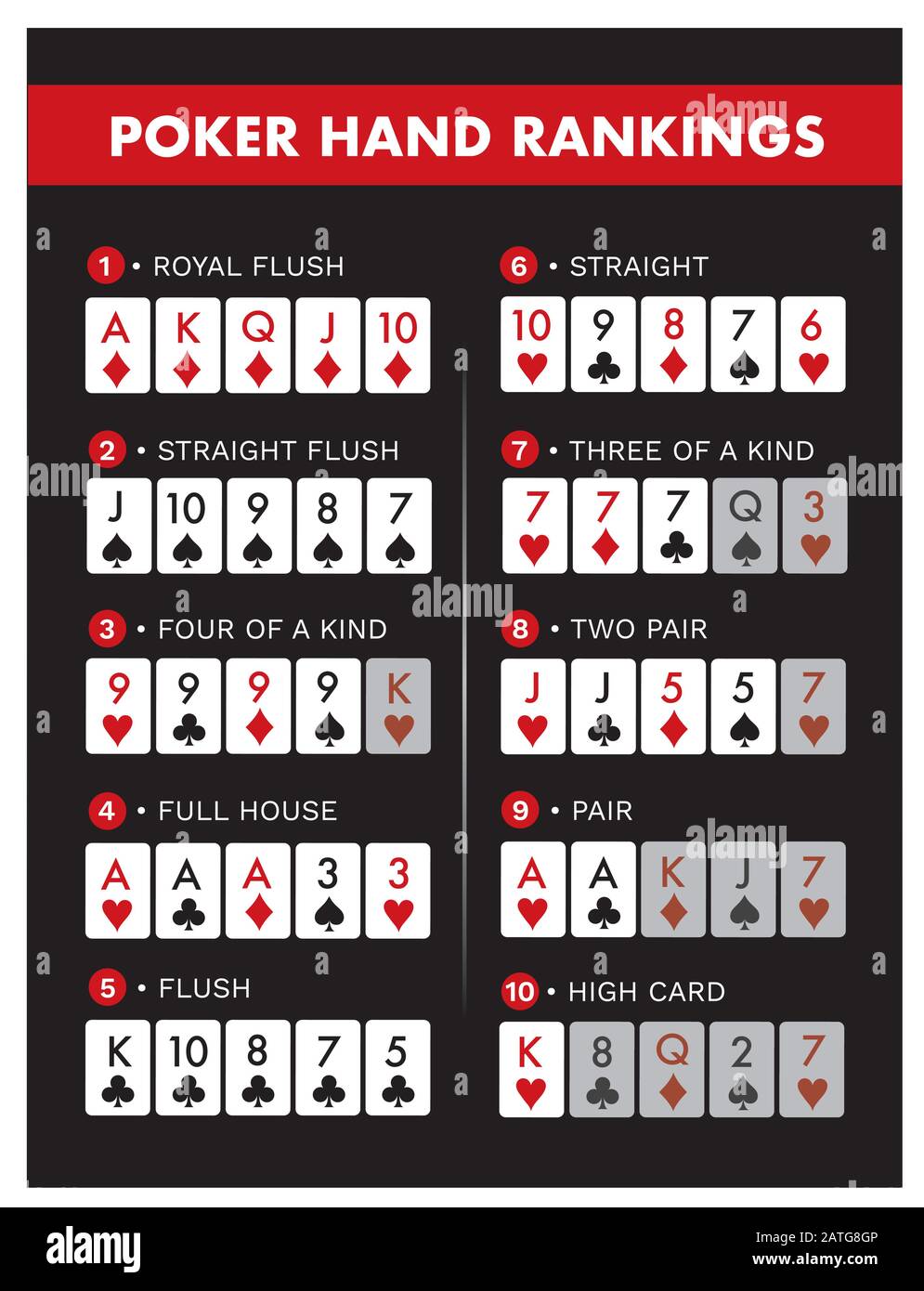
Poker is an exciting game of luck and sheer skills. In Poker, each player creates a set of five playings, i.e., Poker hands. Each Poker hand in the game has a Poker hand ranking that is compared against the competitor’s rank in order to decide who is the winner. In high games like Texas Hold’em and Seven-card stud the Texas Hold’em winning hand emerges as the champion. According to the Poker hand rules, there are ten hand rankings that determine the ultimate winner against the others.
Learning about Poker hands and Texas Holdem winning hands will make you efficient enough to play your best hand and emerge as a champion. Make the most of your skills and opportunities and create a winning Poker hand on your next game at BLITZPOKER.
Royal Flush
A straight from a ten to an ace with all five cards of the same suit. According to Poker hand rules, this is one of the elite suits. This is one of the rarest and greatest Poker hand rankings.
Four of a Kind

Any four cards of the same rank and one side card called kicker. If two players share the same Four of a Kind (on the board), the bigger fifth card (the Kicker) decides who wins the pot.
Flush
Any five cards of the same suit (not consecutive). The highest card of the five determines the rank of the flush. Our example shows an Ace-high flush, which is the highest possible.
Three of a Kind
Any three cards of the same rank. Our example shows three-of-a-kind Aces, with a King and a Queen as side cards – the best possible three of a kind.
One Pair
Any two cards of the same rank. Our example shows the best possible one-pair hand.
Straight Flush
This Poker hand can be any straight, with all five cards of the same suit. The Royal flush is the best possible straight flush where the five cards are the ace, king, queen, jack and ten of a suit.
Full House
Texas Holdem Card Rankings

Any three cards of the same Poker hand rank together with any two cards of the same rank. Our example here, shows Aces full of Kings and it is a bigger full house than Kings full of Aces.
Straight
A Poker hand ranking with any five consecutive cards of different suits. Aces can count as either a high or a low card. Our example shows a five-high straight, which is the lowest possible straight.
Two-pair
Any two cards of the same rank together with another two cards of the same rank. Our example shows the best possible two-pair, Aces and Kings. The highest pair of the two determines the rank of the two-pair.
Texas Holdem Hands Ranking
High-card
Any hand not in the above-mentioned hands. Our example shows the best possible high-card Poker hand.
Introduction
The following table ranks the top hands in an 8-player game. This table assumes that all players stay in until the end.
Explanation of column headings.- Cards: Initial two-card hand.
- Probability of win: Probability that this hand will win, or tie for the win.
- Average win: This is how much the player will win on average, including his own bets, if the player does win. This is less than 8 because sometimes the player will have to split the pot.
- Expected value: This is how many units the player can expected to win (positive) or lose (negative) with this hand. For example if the player had a pair of aces and contibuted $1 to the pot then the player could expect to have a net win of $2.09.
- Probability: Probability of getting this hand to begin with.
- Additive probability: Probability of getting this hand or any stronger hand to begin with.
Initial Hold'em Hands in Rank Order for 8-Player Game
| Cards | Probability of Win | Average Win | Expected Value | Probability | Additive Probability |
| Pair of A's | 39.05% | 7.94 | 2.099 | 0.45% | 0.45% |
| Pair of K's | 33.26% | 7.92 | 1.6328 | 0.45% | 0.9% |
| Pair of Q's | 28.71% | 7.88 | 1.2628 | 0.45% | 1.36% |
| A/K suited | 26% | 7.67 | 0.9953 | 0.3% | 1.66% |
| Pair of J's | 25.13% | 7.84 | 0.9705 | 0.45% | 2.11% |
| A/Q suited | 24.51% | 7.6 | 0.8628 | 0.3% | 2.41% |
| K/Q suited | 23.72% | 7.61 | 0.8043 | 0.3% | 2.71% |
| A/J suited | 23.41% | 7.53 | 0.7616 | 0.3% | 3.02% |
| Pair of T's | 22.32% | 7.79 | 0.7395 | 0.45% | 3.47% |
| A/K unsuited | 22.68% | 7.61 | 0.7264 | 0.9% | 4.37% |
| K/J suited | 22.66% | 7.53 | 0.7073 | 0.3% | 4.68% |
| A/T suited | 22.55% | 7.45 | 0.6803 | 0.3% | 4.98% |
| Q/J suited | 22.1% | 7.52 | 0.6622 | 0.3% | 5.28% |
| K/T suited | 21.87% | 7.47 | 0.633 | 0.3% | 5.58% |
| Q/T suited | 21.38% | 7.45 | 0.5936 | 0.3% | 5.88% |
| J/T suited | 21.26% | 7.44 | 0.5813 | 0.3% | 6.18% |
| A/Q unsuited | 20.98% | 7.51 | 0.5765 | 0.9% | 7.09% |
| Pair of 9's | 19.89% | 7.81 | 0.5527 | 0.45% | 7.54% |
| K/Q unsuited | 20.31% | 7.52 | 0.5281 | 0.9% | 8.45% |
| A/9 suited | 20.28% | 7.39 | 0.4981 | 0.3% | 8.75% |
| A/J unsuited | 19.7% | 7.41 | 0.4607 | 0.9% | 9.65% |
| K/9 suited | 19.5% | 7.42 | 0.4466 | 0.3% | 9.95% |
| A/8 suited | 19.66% | 7.32 | 0.4386 | 0.3% | 10.26% |
| K/J unsuited | 19.1% | 7.43 | 0.4183 | 0.9% | 11.16% |
| Pair of 8's | 18.19% | 7.79 | 0.4164 | 0.45% | 11.61% |
| T/9 suited | 19.18% | 7.38 | 0.4162 | 0.3% | 11.92% |
| Q/9 suited | 19.03% | 7.41 | 0.4107 | 0.3% | 12.22% |
| J/9 suited | 18.96% | 7.4 | 0.4032 | 0.3% | 12.52% |
| A/5 suited | 19.29% | 7.21 | 0.3914 | 0.3% | 12.82% |
| A/7 suited | 19.12% | 7.26 | 0.3883 | 0.3% | 13.12% |
| Q/J unsuited | 18.67% | 7.41 | 0.3832 | 0.9% | 14.03% |
| A/T unsuited | 18.74% | 7.31 | 0.3705 | 0.9% | 14.93% |
| A/4 suited | 18.86% | 7.23 | 0.3638 | 0.3% | 15.23% |
| A/6 suited | 18.62% | 7.22 | 0.3448 | 0.3% | 15.54% |
| A/3 suited | 18.41% | 7.26 | 0.336 | 0.3% | 15.84% |
| K/T unsuited | 18.18% | 7.33 | 0.3328 | 0.9% | 16.74% |
| K/8 suited | 18.05% | 7.33 | 0.3234 | 0.3% | 17.04% |
| Pair of 7's | 16.85% | 7.77 | 0.309 | 0.45% | 17.5% |
| Q/T unsuited | 17.83% | 7.32 | 0.3044 | 0.9% | 18.4% |
| J/T unsuited | 17.86% | 7.3 | 0.3041 | 0.9% | 19.31% |
| A/2 suited | 17.86% | 7.28 | 0.2997 | 0.3% | 19.61% |
| T/8 suited | 17.68% | 7.32 | 0.2949 | 0.3% | 19.91% |
| Q/8 suited | 17.51% | 7.34 | 0.285 | 0.3% | 20.21% |
| J/8 suited | 17.44% | 7.33 | 0.2792 | 0.3% | 20.51% |
| K/7 suited | 17.57% | 7.27 | 0.2777 | 0.3% | 20.81% |
| 9/8 suited | 17.31% | 7.38 | 0.2768 | 0.3% | 21.12% |
| K/6 suited | 17.17% | 7.23 | 0.2413 | 0.3% | 21.42% |
| Pair of 6's | 15.84% | 7.75 | 0.2282 | 0.45% | 21.87% |
| K/5 suited | 16.83% | 7.2 | 0.2117 | 0.3% | 22.17% |
| 8/7 suited | 16.38% | 7.35 | 0.2033 | 0.3% | 22.47% |
| K/4 suited | 16.44% | 7.22 | 0.1869 | 0.3% | 22.78% |
| 9/7 suited | 16.2% | 7.33 | 0.1868 | 0.3% | 23.08% |
| T/7 suited | 16.3% | 7.26 | 0.1827 | 0.3% | 23.38% |
| Q/7 suited | 16.22% | 7.25 | 0.1756 | 0.3% | 23.68% |
| A/9 unsuited | 16.27% | 7.2 | 0.1711 | 0.9% | 24.59% |
| J/7 suited | 16.07% | 7.26 | 0.1662 | 0.3% | 24.89% |
| K/3 suited | 16.06% | 7.26 | 0.1653 | 0.3% | 25.19% |
| Pair of 5's | 14.93% | 7.73 | 0.1542 | 0.45% | 25.64% |
| 7/6 suited | 15.66% | 7.33 | 0.1481 | 0.3% | 25.94% |
| K/2 suited | 15.7% | 7.29 | 0.1446 | 0.3% | 26.24% |
| Q/6 suited | 15.85% | 7.2 | 0.1417 | 0.3% | 26.55% |
| T/9 unsuited | 15.71% | 7.21 | 0.1332 | 0.9% | 27.45% |
| K/9 unsuited | 15.62% | 7.24 | 0.1308 | 0.9% | 28.36% |
| 8/6 suited | 15.37% | 7.3 | 0.1223 | 0.3% | 28.66% |
| Q/5 suited | 15.56% | 7.17 | 0.1158 | 0.3% | 28.96% |
| Pair of 4's | 14.31% | 7.76 | 0.1103 | 0.45% | 29.41% |
| J/9 unsuited | 15.34% | 7.22 | 0.1081 | 0.9% | 30.32% |
| 6/5 suited | 15.12% | 7.32 | 0.1067 | 0.3% | 30.62% |
| Q/9 unsuited | 15.27% | 7.23 | 0.1044 | 0.9% | 31.52% |
| A/8 unsuited | 15.55% | 7.1 | 0.1037 | 0.9% | 32.43% |
| Q/4 suited | 15.16% | 7.2 | 0.0916 | 0.3% | 32.73% |
| 9/6 suited | 14.99% | 7.26 | 0.0891 | 0.3% | 33.03% |
| 5/4 suited | 14.74% | 7.32 | 0.0789 | 0.3% | 33.33% |
| T/6 suited | 15.03% | 7.18 | 0.0785 | 0.3% | 33.63% |
| Pair of 3's | 13.83% | 7.8 | 0.0782 | 0.45% | 34.09% |
| 7/5 suited | 14.74% | 7.29 | 0.0741 | 0.3% | 34.39% |
| Q/3 suited | 14.81% | 7.24 | 0.0721 | 0.3% | 34.69% |
| J/6 suited | 14.92% | 7.17 | 0.0691 | 0.3% | 34.99% |
| Pair of 2's | 13.49% | 7.83 | 0.057 | 0.45% | 35.44% |
| Q/2 suited | 14.46% | 7.28 | 0.0524 | 0.3% | 35.75% |
| A/5 unsuited | 15.1% | 6.95 | 0.0489 | 0.9% | 36.65% |
| A/7 unsuited | 14.94% | 7.01 | 0.0473 | 0.9% | 37.56% |
| J/5 suited | 14.66% | 7.13 | 0.0454 | 0.3% | 37.86% |
| 6/4 suited | 14.07% | 7.33 | 0.0306 | 0.3% | 38.16% |
| 8/5 suited | 14.22% | 7.24 | 0.0303 | 0.3% | 38.46% |
| J/4 suited | 14.28% | 7.16 | 0.0222 | 0.3% | 38.76% |
| A/4 unsuited | 14.63% | 6.96 | 0.0184 | 0.9% | 39.67% |
| 5/3 suited | 13.71% | 7.33 | 0.0049 | 0.3% | 39.97% |
| J/3 suited | 13.92% | 7.2 | 0.0023 | 0.3% | 40.27% |
| A/6 unsuited | 14.39% | 6.95 | 0 | 0.9% | 41.18% |
| T/8 unsuited | 14.07% | 7.11 | -0.0002 | 0.9% | 42.08% |
| K/8 unsuited | 14.01% | 7.09 | -0.0059 | 0.9% | 42.99% |
| 9/5 suited | 13.81% | 7.19 | -0.0066 | 0.3% | 43.29% |
| T/5 suited | 13.98% | 7.09 | -0.0092 | 0.3% | 43.59% |
| A/3 unsuited | 14.15% | 6.99 | -0.0111 | 0.9% | 44.49% |
| 9/8 unsuited | 13.73% | 7.18 | -0.0137 | 0.9% | 45.4% |
| J/2 suited | 13.59% | 7.24 | -0.0154 | 0.3% | 45.7% |
| 7/4 suited | 13.48% | 7.28 | -0.0187 | 0.3% | 46% |
| J/8 unsuited | 13.68% | 7.11 | -0.0278 | 0.9% | 46.91% |
| T/4 suited | 13.64% | 7.11 | -0.0304 | 0.3% | 47.21% |
| Q/8 unsuited | 13.6% | 7.11 | -0.0331 | 0.9% | 48.11% |
| 4/3 suited | 13.09% | 7.37 | -0.0351 | 0.3% | 48.42% |
| T/3 suited | 13.3% | 7.15 | -0.049 | 0.3% | 48.72% |
| A/2 unsuited | 13.54% | 7.01 | -0.0507 | 0.9% | 49.62% |
| K/7 unsuited | 13.48% | 7 | -0.0557 | 0.9% | 50.53% |
| 6/3 suited | 12.85% | 7.33 | -0.0587 | 0.3% | 50.83% |
| 8/4 suited | 12.98% | 7.22 | -0.0626 | 0.3% | 51.13% |
| T/2 suited | 12.95% | 7.19 | -0.0682 | 0.3% | 51.43% |
| 5/2 suited | 12.5% | 7.33 | -0.0839 | 0.3% | 51.73% |
| 8/7 unsuited | 12.79% | 7.13 | -0.0877 | 0.9% | 52.64% |
| 9/4 suited | 12.71% | 7.16 | -0.0908 | 0.3% | 52.94% |
| K/6 unsuited | 13.02% | 6.94 | -0.0972 | 0.9% | 53.85% |
| 4/2 suited | 12.08% | 7.38 | -0.1079 | 0.3% | 54.15% |
| 7/3 suited | 12.25% | 7.27 | -0.1101 | 0.3% | 54.45% |
| 9/3 suited | 12.37% | 7.19 | -0.1108 | 0.3% | 54.75% |
| 9/7 unsuited | 12.54% | 7.09 | -0.1114 | 0.9% | 55.66% |
| T/7 unsuited | 12.55% | 6.98 | -0.1238 | 0.9% | 56.56% |
| 9/2 suited | 12.03% | 7.24 | -0.1287 | 0.3% | 56.86% |
| K/5 unsuited | 12.65% | 6.88 | -0.1298 | 0.9% | 57.77% |
| 7/6 unsuited | 12.08% | 7.09 | -0.1433 | 0.9% | 58.67% |
| 8/3 suited | 11.88% | 7.19 | -0.1452 | 0.3% | 58.97% |
| 3/2 suited | 11.49% | 7.43 | -0.1454 | 0.3% | 59.28% |
| 6/2 suited | 11.62% | 7.32 | -0.1492 | 0.3% | 59.58% |
| Q/7 unsuited | 12.22% | 6.95 | -0.1511 | 0.9% | 60.48% |
| J/7 unsuited | 12.18% | 6.97 | -0.1514 | 0.9% | 61.39% |
| K/4 unsuited | 12.21% | 6.9 | -0.1579 | 0.9% | 62.29% |
| 8/2 suited | 11.57% | 7.23 | -0.163 | 0.3% | 62.59% |
| 8/6 unsuited | 11.7% | 7.04 | -0.1767 | 0.9% | 63.5% |
| K/3 unsuited | 11.79% | 6.94 | -0.1818 | 0.9% | 64.4% |
| 6/5 unsuited | 11.51% | 7.07 | -0.1865 | 0.9% | 65.31% |
| Q/6 unsuited | 11.81% | 6.87 | -0.189 | 0.9% | 66.21% |
| 7/2 suited | 11.19% | 7.24 | -0.1897 | 0.3% | 66.52% |
| K/2 unsuited | 11.41% | 6.98 | -0.2035 | 0.9% | 67.42% |
| 5/4 unsuited | 11.12% | 7.06 | -0.2149 | 0.9% | 68.33% |
| 9/6 unsuited | 11.22% | 6.97 | -0.2182 | 0.9% | 69.23% |
| Q/5 unsuited | 11.46% | 6.82 | -0.2191 | 0.9% | 70.14% |
| 7/5 unsuited | 11.07% | 7 | -0.2249 | 0.9% | 71.04% |
| T/6 unsuited | 11.18% | 6.83 | -0.2361 | 0.9% | 71.95% |
| Q/4 unsuited | 11.03% | 6.84 | -0.2454 | 0.9% | 72.85% |
| J/6 unsuited | 10.94% | 6.8 | -0.2562 | 0.9% | 73.76% |
| 6/4 unsuited | 10.39% | 7.05 | -0.2677 | 0.9% | 74.66% |
| Q/3 unsuited | 10.63% | 6.88 | -0.2679 | 0.9% | 75.57% |
| 8/5 unsuited | 10.47% | 6.92 | -0.2757 | 0.9% | 76.47% |
| J/5 unsuited | 10.64% | 6.73 | -0.2837 | 0.9% | 77.38% |
| Q/2 unsuited | 10.26% | 6.93 | -0.289 | 0.9% | 78.28% |
| 5/3 unsuited | 10.04% | 7.04 | -0.2936 | 0.9% | 79.19% |
| J/4 unsuited | 10.22% | 6.76 | -0.3091 | 0.9% | 80.09% |
| 9/5 unsuited | 9.97% | 6.82 | -0.3206 | 0.9% | 81% |
| 7/4 unsuited | 9.73% | 6.95 | -0.3238 | 0.9% | 81.9% |
| T/5 unsuited | 10.06% | 6.65 | -0.3308 | 0.9% | 82.81% |
| J/3 unsuited | 9.83% | 6.81 | -0.3312 | 0.9% | 83.71% |
| 4/3 unsuited | 9.38% | 7.08 | -0.336 | 0.9% | 84.62% |
| J/2 unsuited | 9.46% | 6.86 | -0.3512 | 0.9% | 85.52% |
| T/4 unsuited | 9.66% | 6.67 | -0.3554 | 0.9% | 86.43% |
| 6/3 unsuited | 9.08% | 7 | -0.3642 | 0.9% | 87.33% |
| 8/4 unsuited | 9.12% | 6.84 | -0.3766 | 0.9% | 88.24% |
| T/3 unsuited | 9.28% | 6.72 | -0.3767 | 0.9% | 89.14% |
| 5/2 unsuited | 8.73% | 7 | -0.3892 | 0.9% | 90.05% |
| T/2 unsuited | 8.93% | 6.76 | -0.3962 | 0.9% | 90.95% |
| 9/4 unsuited | 8.76% | 6.71 | -0.4126 | 0.9% | 91.86% |
| 4/2 unsuited | 8.3% | 7.06 | -0.4138 | 0.9% | 92.76% |
| 7/3 unsuited | 8.41% | 6.88 | -0.4219 | 0.9% | 93.67% |
| 9/3 unsuited | 8.4% | 6.74 | -0.4338 | 0.9% | 94.57% |
| 9/2 unsuited | 8.04% | 6.8 | -0.4531 | 0.9% | 95.48% |
| 3/2 unsuited | 7.67% | 7.12 | -0.4543 | 0.9% | 96.38% |
| 6/2 unsuited | 7.79% | 6.94 | -0.46 | 0.9% | 97.29% |
| 8/3 unsuited | 7.96% | 6.73 | -0.4646 | 0.9% | 98.19% |
| 8/2 unsuited | 7.61% | 6.77 | -0.4844 | 0.9% | 99.1% |
| 7/2 unsuited | 7.28% | 6.77 | -0.5073 | 0.9% | 100% |
| Total | 7.2 | 0 | 100% |
Methodology
This table is the result of a random simulation of 25,729,704,000 games and assumes all players stay in until the end of the hand.
The following table shows my power rating for each initial 2-card hand in a 8-player game. The numbers are on a 0 to 40 scale. Basically, you should only play hands that are dark green, blue, or purple. Of course you should be more be more liberal in late position and picky in early position.
Texas Holdem Hand Chart
Use the top table if you have a pair, the middle table if your cards are suited, and the bottom table if your cards are unsuited. Except for a pair,look up your high card along the left and your low card along the top.